PBTK model for dioxin

This page is a encyclopedia article. The page identifier is Op_en6739
Moderator:Heta (see all)
Upload data {{#opasnet_base_link:Op_en6739}}

The text on this page has been taken from an equivalent page of the IEHIAS-project. For the main article about dioxins, see Dioxin. For the main method article about kinetic models of dioxin, see Human PBPK model for dioxin.

This model relates to physiologically based toxicokinetics modelling process. The presented PBTK model allows to simulate ingestion exposures to 2,3,7,8-tétra-chloro-p-dioxin (TCDD) for a woman over her whole life. The model computes various measures of internal dose as a function of time. The superposition of a peak exposure to a time-varying background intake can be described. The model equations are solved dynamically (by numerical integration) with the MCSim simulation package to give a good precision both on short-term and long-term scales. The body mass and the volume of the various body tissues change with the age of the simulated individual.

Model description

Purpose

This model relates to physiologically based toxicokinetics modelling process. The presented PBTK model allows to simulate ingestion exposures to 2,3,7,8-tétra-chloro-p-dioxin (TCDD) for a woman over her whole life. TCDD is a persistent chemical found in trace amounts all over the globe. It accumulates in animal fat all along the trophic chain. Human exposure to TCDD is therefore almost unavoidable, even if in trace amounts. TCDD has multiple effects on health.

Boundaries

It is related to woman exposure through food.

Input

Input data have to be written directly on the webpage. Data consist in: background rates, for different periods of life, beginning, end and level of peak exposure.

Output

Output data are the concentrations in different parts of the body (blood, liver, fat, well-perfused tissues and poorly-perfused tissues) as a function of time.

Description of processes modelled and of technical details

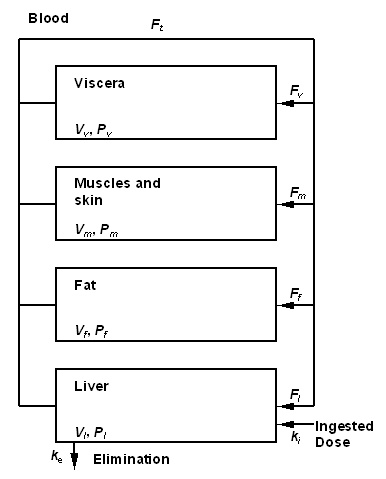
Figure 1 : Toxicokinetic model used to describe TCDD toxicokinetic in the human body (d'après (Van der Molen et al. 1996)). compartments are characterized with volume V and partition coefficient P. Exchanges between are governed by blood flows, F. Elimination is assumed proportional to the elimination constant, ke. The set value of each parameter is given in Table 1. The ingested quantity by unit of time ki (en ng/min), is determined by the exposure scenario.

Table 1 : Numerical values of the parameters of the toxicokinetic model for TCDD in the woman.

Parameter(a)	Symbol	Numerical Value
Ventilation rate	Fp	8,0
Blood over air ventilation rate	R	1,14
Blood flow rates
Fat	Ff	0,09
Liver	Fl	0,24
Muscles and skin	fm	0,18
Viscera	fv	– (b)
Volumes
Total body volume	Vt	– (c)
Fat	Vf	– (c)
Liver	Vl	– (c)
Muscles and skin	Vm	– (c)
Viscera	Vv	– (c)
Partition Coefficient
Fat	Pf	300
Liver	Pl	25
Muscles and skin	Pm	4
Viscera	Pv	10
Elimination constant	ke	8,45´;10-8 (d)

(a) Units : volumes (L), blood flow (L/min), et elimination constant (min-1).

(b) Blood flow rate to viscera is calculated by difference between 1 and the sum of blood flow rates toward the other compartments.

(c) Volumes evolve with time.

(d) Corresponds to a half-life of 15,6 years.

Figure 1 gives a graphical representation of the model used. Only ingestion exposure is described in this model (the totality of the exposure dose is assumed to be absorbed). The TCDD is supposed to be distributed into different compartments of the body : blood, fat, muscles and skin. The original formulation of van der Molen and coll. (Van der Mollen et al. 1996) regards all these compartments as being with balance in an instantaneous way. This assumption is acceptable only if slow evolutions of absorption are the limiting factor of the kinetics of the product. Since the simulation of a short peak of exposure interests us, we developed a traditional dynamic formulation (Gerlowski et al. 1983), specifies at the same time on short scales of time and the long-term.

Model equations

Equations defining the proposed model are the following:

For quantities of TCDD in fat, viscera, muscle and skin, and liver:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\delta Q_f}{\delta t} = f_f \cdot F_t \Bigl( C_{art} - \frac{Q_f}{V_f \times P_f} \Bigr) \qquad (1) }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\delta Q_v}{\delta t} = f_v \cdot F_t \Bigl( C_{art} - \frac{Q_v}{V_v\times P_v} \Bigr) \qquad (2) }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\delta Q_m}{\delta t} = f_m \cdot F_t \Bigl( C_{art} - \frac{Q_m}{V_m\times P_m} \Bigr) \qquad (3) }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\delta Q_l}{\delta t} = f_l \cdot F_t \Bigl( C_{art} - \frac{Q_l}{V_l\times P_l} \Bigr) - k_e \times Q_l + k_i \qquad (4) }

The arterial concentration is calculated by:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle C_{art} = \Bigl(\frac{f_f \times Q_f}{V_f \times P_f} + \frac{f_v \times Q_v}{V_v \times P_v} + \frac{f_m \times Q_m}{V_m \times P_m} + \frac{f_f \times Q_l}{V_f \times P_l}\Bigr) \qquad (5) }

Cardiac output F_t is proportional to the ventilation rate Fp:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_t = 0.7 \times F_p \times R \qquad (6) }

The body volume evolves as a function of age:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_t = 0.1959 \times t - \frac{57.497}{(1+4.617 \times exp(-0.572 \times (t-11.33)))^{1/4.617}} \qquad (7) }

The volume of fat, viscera, liver also evolve as a function of age (Van der Molen et al. 1996):

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_g = \begin{cases} 0.5+4.5 \times \frac{t}{10}, \qquad\text{if }t\ge 10\text{,}\\ 0.5+4.5+8\times\frac{t-10}{15}, \qquad\text{if }10 \le t \le 15\\0.5+4.5+8+17\times\frac{t-15}{55}, \qquad\text{if }t \ge15\text{.}\end{cases} \qquad (8) }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_v = \frac{6.095}{(1+4.617 \times exp(-0.3937 \times (t-6.5582)))^{1/4.617}} \qquad (9) }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_f = \frac{1.758}{(1+4.617 \times exp(-0.3309 \times (t-12.478)))^{1/4.617}} \qquad (10) }

Volume of "muscles and skin" compartment is calculated as the difference between 90% of the total body volume (because bones are not included) and the other compartments:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_m = 0.9 \times V_t - V_f - V_v - V_l \qquad (11) }

Units used are : quantities of TCDD are expressed in ng, TCDD concentrations in ng/L, age in years volumes in liter, flows in L/min, the elimination constant in min-1, the ingested quantity by unit of time in ng/min. The body density is assumed equal to 1.

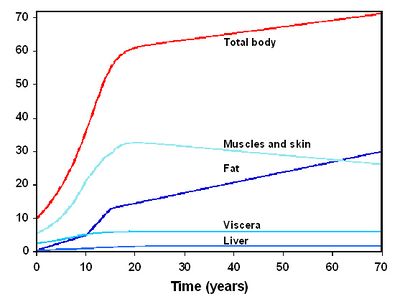
Figure 2 presents the temporal evolution of these parameters for a woman (the evolution is overall similar for a man). The reference averaged values used for the parameters not evolving with time are given in Table 1. The equations of the model were coded using the MCSim software (Bois et al. 1997).

Figure 2 : Temporal evolution of volumes for the woman (Van der Molen et al. 1996).

Rationale

The proposed model is based on a previous one proposed by van der Mollen and colleagues in 1996 (Van der Mollen et al. 1996). The model computes various measures of internal dose as a function of time. The superposition of a peak exposure to a time-varying background intake can be described. All ingested TCDD is supposed to be absorbed. TCDD is supposed to distribute between blood, fat, muscles and skin, and viscera. The model equations are solved dynamically (by numerical integration) with the MCSim simulation package to give a good precision both on short-term and long-term scales. The body mass and the volume of the various body tissues change with the age of the simulated individual.

References

• Bois, F. Y. (2003). "Modélisation toxicocinétique de la concentration sanguine de 2,3,7,8-tetrachloro-p-dioxine après ingestion chez la femme." Environnement, Risques et Santé 2(1).
• Bois, F. Y. and D. Maszle (1997). "MCSim: a simulation program." Journal of Statistical Software 2(9)
• Gerlowski, L. E. and R. K. Jain (1983). "Physiologically based pharmacokinetic modeling: principles and applications." Journal of Pharmaceutical Sciences 72: 1103-1127.
• Van der Molen, G. W., S. A. L. M. Kooijman and W. Slob (1996). "A generic toxicokinetic model for persistent lipophilic compounds in humans: an application to TCDD." Fundamental and Applied Toxicology 31: 83-94.

See also

• Contact person: Frédéric Y. Bois (frederic.bois [at] ineris.fr
• Time required for a typical run: Typically one second
• Degree of mastery: Basic user / Expert user
• Intellectual property rights (who is allowed to access or use the model?): Free software.
• Tool website: [1] and [2]