Life tables

The DALY calculation tool designed for use in association with the INTARESE methodology uses life tables to calculate years of life lost (YLL). The estimation of burden of disease in disability-adjusted life years (DALYs) requires data on mortality ("quantity of life lost") and morbidity ("quality of life lost"). The burden of disease due to mortality consists of the total number of years of life lost (YLLs). This can be calculated for any health outcome as the age-specific mortality multiplied by age-specific life expectancy based on standard life-table analysis.

Introduction

Figure 1 - An example of an interim life table for England, 2004. From the UK Office of National Statistics website.

Life tables (or mortality tables or actuarial tables) are tables that indicate the probability that a person in a given population at an certain age will die before their next birthday. The first "life table" was drawn up in 1603 by John Graunt­ in his Bills of Mortality. They are generally drawn up separately by sex, and may also be drawn up for subsets of a population, taking into account other factors (e.g. ethnicity etc.). These tables are used in several fields, including epidemiology, biology and actuarial science (insurance) to calculate a number of other statistics, including:

• the probability of surviving any particular year of age;
• remaining life expectancy for people at different ages;
• the proportion of the original birth cohort still alive; and
• estimates of a cohort's longevity characteristics.

In the context of integrated environmental health assessment (i.e. the INTARESE method), life tables and the statistics derived from them may be used in the valuation of health impacts.

Using life tables to calculate population statistics

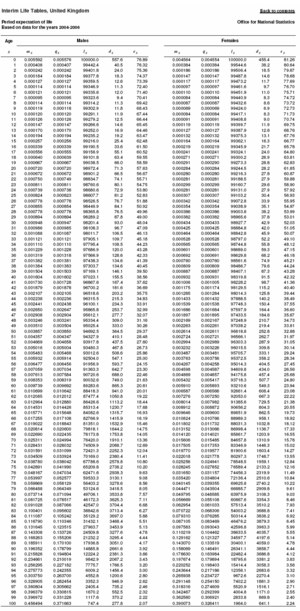
Figure 1 shows an interim life table for the UK for 2004-6. The notation used in the figure (and commonly used in life tables) is as follows:

• m_x = is the central rate of mortality, defined as the number of deaths at age x last birthday in the three year period to which the Interim Life Table relates divided by the average population at that age over the same period.
• q_x = is the mortality rate between age x and (x+1), that is the probability that a person aged x exact will die before reaching age (x +1).
• l_x = is the number of survivors to exact age x of 100,000 live births of the same sex who are assumed to be subject throughout their lives to the mortality rates experienced in the three year period to which the Interim Life Table relates.
• l_x = is the number dying between exact age x and (x+1) described similarly to l_x, that is d_x=l_x-l_x+1
• e_x = is the average period expectation of life at exact age x, that is the average number of years that those aged x exact will live thereafter based on the mortality rates experienced in the three year period to which the Interim Life Table relates.

Graduated life tables vs. interim life tables

Interim life tables may be produced to provide statistics on period life expectancy by age and sex using extrapolation from graduated life table data. For example, in the UK interim life tables are released annually; these are based on the population estimates and deaths data for a period of three consecutive years. These interim life tables are based on fully graduated life tables, which are produced every decade and are based on decennial population censuses.

Types of life expectancy

There are two main types of life expectancy:

• period life expectancy
• cohort life expectancy

Period life expectancy (at a given age for a certain area) is the average years a person would live, if she/he experienced that area's age-specific mortality rates for that time period throughout the whole of his or her life. Importantly, this does not make allowances for later actual or projected changes in mortality (death rates in an area are, in reality, subject to change with time). This means that period life expectancy does not provide the actual average number of years someone could expect to live. Cohort life expectancies, however, are estimated using age-specific mortality rates. These rates allow known or projected changes in mortality in later years. These estimates of life expectancy are considered to be closer to the actual average years a person of a given age can expect to live. As an example, period life expectancy at age 65 in 2000 would be calculated using the mortality rate for that age group (65) in the year 2000, for age 66 in 2000, for age 67 in 2000 etc. Cohort life expectancy, however, at the same age would be using the mortality rates for age 65 in 2000, for age 66 in 2001, for age 67 in 2002, and so on.

Period life expectancy answers the question ‘For a group of people aged x in a given year, how long would they live, on average, if they experienced the age-specific mortality rates above age x of the period in question over the course of their remaining lives?’ The cohort life expectancy answers the question ‘For a group of people aged x in a given year, how long would we expect them to live, on average, if they experienced the actual or projected future age-specific mortality rates not from the given year but from the series of future years in which they will actually reach each succeeding age if they survive?’ If mortality rates at age x and above are projected to decrease in future years, the cohort life expectancy at age x will be greater than the period life expectancy at age x.

Methodologies used to calculate life expectancy at birth

Several methods may be used to calculate life expectancy at birth. For example, the UK Office for National Statistics (ONS) uses one of two methods proposed by Chiang. Another commonly used method has been developed by Silcocks.

Further reading, resources and links

• Life tables for WHO member states (including downloadable .csv files)
• ONS Report: "Life expectancy at birth: methodological options for small populations"
• ONS template for calculating life expectancy and standard error.
• Lecture on life table analsis (Life Table Analysis: Charles M. Friel Ph.D., Criminal Justice Center, Sam Houston State University) - word document